This task includes an experimental GeoGebra worksheet, with the intent that instructors might use it to more interactively demonstrate the relevant content material.

This task emphasizes the expectation that students know linear functions grow by constant differences over equal intervals and exponential functions grow by constant factors over equal intervals.

This task has students explore the relationship between the three parameters a, h, and k in the equation f(x)=a(xh)2+k and the resulting graph.

The purpose of this task is to use the definition of rotations in order to find the center and angle of rotation given a triangle and its image under a rotation.

The purpose of this task is to study the impact of translations on triangles.

The goal of this task is to get students to focus on the shape of the graph of an equation and how this changes depending on the sign of the exponent and on whether the exponential is in the numerator or denominator.

This task asks students to interpret the relevant parameters in terms of the real-world context and describe exponential growth.

This problem assumes students have completed several preliminary tasks about the fact that linear functions change by equal differences over equal intervals.

This Illustrative Mathematics task encourages students to reason quantitatively about the structure of the expression.

The purpose of this task is to help students see manipulation of expressions as an activity undertaken for a purpose. The expression arises in physics as the reciprocal of the combined resistance of two resistors in parallel. However, the context is not explicitly considered here.
Variation 1 of this task presents a related more complex expression already in the correct form to answer the question.

A parachute is made from thin, lightweight fabric, support tapes and suspension lines. The lines are usually gathered through cloth loops or metal connector links at the ends of several strong straps called risers. The risers in turn are attached to the harness containing the load.

The principal purpose of this task is to probe students' ability to correlate symbolic statements about a function using function notation with a graph of the function, and to interpret their answers in terms of the quantities between which the function describes a relationship.

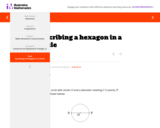
This task is primarily for instructive purposes but can be used for assessment as well. Parts (a) and (b) are good applications of geometric constructions using a compass and could be used for assessment purposes but the process is a bit long since there are six triangles which need to be constructed.

This task implements many important ideas from geometry including trigonometric ratios, important facts about triangles, and reflections. As a result, it is recommended that this task be undertaken relatively late in the geometry curriculum.

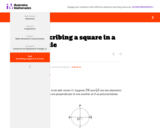
This task provides an opportunity for students to apply triangle congruence theorems in an explicit, interesting context.

Open Middle provides math problems that have a closed beginning, a closed end, and an open middle. This means that there are multiple ways to approach and ultimately solve the problems. Open middle problems generally require a higher Depth of Knowledge than most problems that assess procedural and conceptual understanding.

Given two bank interest rate scenarios, students will compare returns, write an expression for a balance, and create a table of values for the balances.

Students will use the graph (for example, by marking specific points) to illustrate the statements in (a) and (d). If possible, label the coordinates of any points you draw.